D’Arcy Wentworth Thompson and Mathematics
Over the summer, two undergraduate students Alice Gowenlock and Indre Tuminauskaite, took part in a research internship funded by the Strathmartine Trust and the School of Mathematics and Statistics. The internship focused on the mathematical knowledge of D’Arcy Wentworth Thompson. In this blog Alice and Indre share what they found in the D’Arcy Thompson archive, held by the Library’s Special Collections Division.
***
How did a person, who claimed not to know any mathematics, write one of the first mathematical biology books?
D’Arcy Wentworth Thompson is the writer of the revolutionary On Growth and Form, an influential book which explored the mathematical explanations for organic forms. He was an accomplished man in many fields; from biology and mathematics to languages and literature. It was his ability to link his knowledge of these subjects together which really made On Growth and Form the classic that it is.
Last year’s centenary conference about On Growth and Form raised questions about D’Arcy’s mathematical knowledge. With On Growth and Form being mainly a book on mathematical ideas, we were set the interesting task of exploring how much maths he actually knew and how he gained this knowledge.
D’Arcy was primarily a biologist, moving to Cambridge to study zoology after just two years of medical school in Edinburgh. After his education, he became Professor of Biology at University College, Dundee in 1884 and later Chair of Natural History at the University of St Andrews in 1917. The acquisition of his biological knowledge, as seen in On Growth and Form, is easy to see through his formal education but what about the mathematical skills needed to write about such complicated and ground-breaking subjects?
We were keen to see how D’Arcy’s correspondence could shed light on the answers to these questions. Special Collections hosts over 30,000 documents relating to D’Arcy, given to the University by Ruth, one of his three daughters. They include correspondence with mathematicians, physicists and engineers; including William Peddie, Claxton Fidler, E H Neville and many others.
- D’Arcy Wentworth Thompson
- Caricature of D’Arcy Wentworth Thompson (ms46331/3)
On Growth and Form encompasses many areas of mathematics but there are a few key topics which are of particular importance.
The chapter on the ‘Form of Cells’ explains the effects of different forces such as surface tension on the form of cells and considers the similarity between the forms of single cell organisms and Plateau’s Surfaces of Revolution. This section was heavily influenced by the work of C R Darling, a physicist who published his book, Liquid Drops and Globules in 1914 and made improvements to Plateau’s experiments. D’Arcy was very interested in how soap-bubbles, liquid drops and oil globules related to cell forms and asked Darling if he could use some of his ideas.
It is also thought that D’Arcy acquired some of his scientific and mathematical knowledge during his two years at Edinburgh University. We discovered that one particular influence was physicist P G Tait, who is mentioned in On Growth and Form for stressing the importance of the mathematical aspect of physics. We were able to examine D’Arcy’s class exam scripts from Tait’s class at Edinburgh (ms47908, dated 1877) in Special Collections. His solutions clearly show his talent for science and several answers relate very closely to the ideas discussed in On Growth and Form, for example on the form of soap-bubbles.
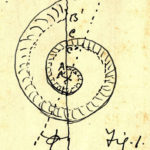
Thompson is recognised for his work relating the logarithmic spiral to natural forms. In this type of spiral the distance between the whorls continually increases. This is due to twisting at a constant rate but growing at a constant acceleration. It can be seen in spiders’ webs, rams’ horns and the shells of molluscs for example. We uncovered correspondence between D’Arcy and Scottish physicist and applied mathematician William Peddie on the topic of these spirals and how they relate to actual forms. In one of the key letters (ms45791), Peddie describes in detail the coiling of such a spiral and the mathematics behind it.
- D’Arcy’s class exam scripts from Tait’s class at Edinburgh (ms47908, dated 1877)
- Peddie’s sketch of a logarithmic spiral
During our research internship we had the opportunity to visit the D’Arcy Thompson Zoology Museum in Dundee, which houses many interesting specimens collected by D’Arcy, as well as visiting the Bell Pettigrew Museum in St Andrews. It was fascinating to see some of the artefacts that he used to demonstrate his ideas close up and we would highly recommend giving both museums a visit. We especially enjoyed seeing the examples of the logarithmic spiral in specimens such as the nautilus shell, walrus tusks and whale teeth.
- Alice Gowenlock outside the D’Arcy Thompson Zoology Museum
- Form of soap bubbles
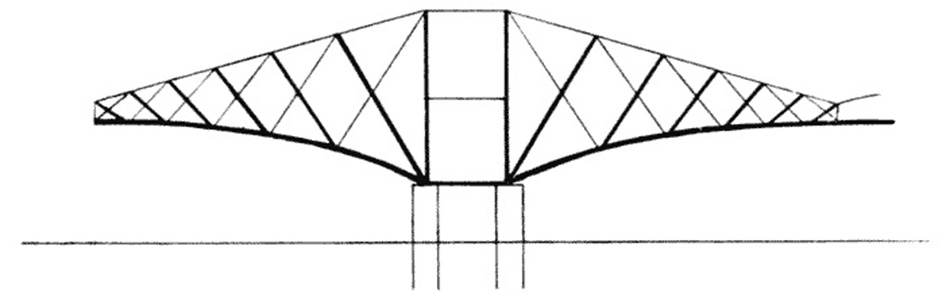
One of D’Arcy’s correspondents who possibly had the most influence on On Growth and Form was British engineer Claxton Fidler. In the correspondence between them, Fidler discusses, in detail, the comparisons between the bone skeleton and the framework of a steel bridge and states that the structure of an animal skeleton is most comparable to the main girder of a double-armed cantilever bridge (such as the Forth Bridge). The analogy of the bridge stems from the idea that both structures rely on an alternately rigid and flexible system. Thompson credits Fidler in the preface of the book for his vast contribution, especially to the chapter on mechanical efficiency.
One of the most recognisable and celebrated parts of On Growth and Form is the Theory of Transformations section, where forms of related species are compared through mathematical transformations. It involves drawing an outline of an animal on a Cartesian grid and submitting the grid to a coordinate transformation. Thompson did not master this section alone and received help on the transformations from others including maths lecturer John Marshall.
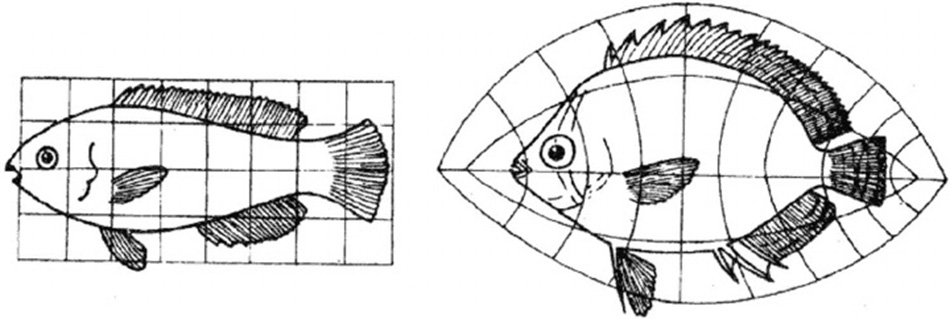
In On Growth and Form, D’Arcy deals with the transformations in 2D, however there is a small section at the end of this chapter which mentions the use of 3-dimensional coordinates. It is on this subject that we discovered correspondence between D’Arcy and English mathematician E H Neville, a specialist in Geometry, who provided Thompson with an explanation of fish transformations in 3D. D’Arcy had trouble understanding this and said, “…this extended theme I have not attempted to pursue, and it must be left to other times, and to other hands.”
Exploring the life and work of D’Arcy Thompson through his vast correspondence and On Growth on Form has been fascinating. We have discovered that, despite writing one of the most influential books in Mathematical Biology, D’Arcy’s knowledge of mathematics was indeed rather limited. It was through communication with a network of other scientists and mathematicians that D’Arcy’s ideas blossomed and brought together two areas of science that are now inextricably linked, today more than ever before.
To find out more about D’Arcy Thompson and the mathematics behind On Growth and Form click here.
Alice Gowenlock
Indre Tuminauskaite





[…] on from last year’s project with Alice and Indre, interns from the School of Mathematics, this year we had two more interns […]
Is there a book on Thompson and Mathematics? Great Thanks